|
Thin
film chip resistors are physical structures with high power dissipation
capability, small size, optimal and stable operating characteristics at
frequencies as high as 20 GHz. These chip resistors are design to be used in
microwave hybrid circuits for high speed transimpedance amplifiers.
Even
a component as simple as a resistor exhibit complex behavior at high frequencies
because of different parasitic effects.
The
simplest RF lumped circuit model for a physical resistor might include a series
inductance due to the metal electrodes and a parallel capacitance formed as a
result of the ceramic dielectric on which the resistor is constructed. The
presence of parasitic inductance and capacitance causes the impedance to depart
from a pure, frequency-independent resistance.
Very
low values of resistance suffer from an early impedance increase, starting
approximately at a frequency where the reactance of the series inductance
becomes significant compared with the resistance. Similarly, high resistance
suffer a premature impedance decrease from the shunt capacitance. The
frequency range over which the impedance remains roughly constant is maximized
for some intermediate resistance value.

If the resistance equals Zo, the bandwidth Fmax over which the impedance remains
approximately constant is given by resonant frequency of the LC combination. The
smaller the LC product, the greater the frequency range over which the resistor
looks approximately resistive.
In
order to analyze the behavior of the thin film resistors at high frequencies we
use SONNET software for high-frequency electromagnetic analysis Em. It use a
modified method of moments analysis based on Maxwell's equations to perform a
true three dimensional current analysis of predominantly planar structures. We
perform a "full-wave" analysis for thin film resistor geometry, taking into
account all possible coupling mechanisms. To model a thin film resistor we use a
circuit containing two microstrip transmission lines with the thin film resistor
in the middle. The port discontinuity and the transmission line effect are
removed from the analysis results by a de-embedding process. The analysis starts
by subdividing the circuit metallization into small rectangular subsections. Em
automatically places small subsections in critical areas where current density
is changing rapidly, but allows larger subsections in less critical areas, where
current density is smooth or changing slowly. The automatic algorithm may be
modified in order to obtain an analysis time or the required level of accuracy.
On the two transmission lines, conductors which are nearly lossless, current
travels primarily along the outer edges of the conductor.Thus, enabling edge
mesh for these conductors can reduce simulation time with little loss in
accuracy.
The
results of RG series thin film resistors analyses indicate some general rules:
-
Small chips like RG1020, RG1025 and RG2000 may be used at a high frequency due
to their lower shunt capacitance.
-
RG1020,RG1025, RG1421 and RG2040 exhibit an increase of the impedance with
frequency;for the ratio Lr/Wr resistance length /resistance width up to 0.25 for
RG1020 and RG1025 and up to 0.5 for RG1215 and RG1421 the impedance increase is
not more than 10%.(fig.1)
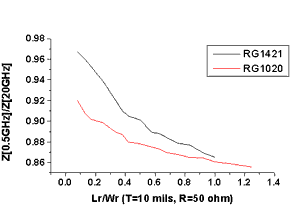
Fig.1. Ratio Z at 0.5 GHz/Z at 20 GHz vs. ratio Lr/Wr for
RG1020 and RG1421. |
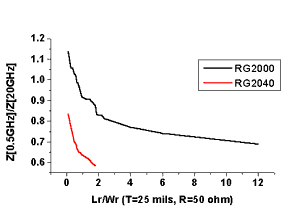
Fig.2.Ratio Z at 0.5GHz/Z at 20GHz for RG2000 and RG2040.
The
S11 reflection coefficient remains under -20dB for the most geometries of the RG
series but increases when the ratio Lr/Wr is increased.(Fig.3,4)

Fig.3. The S11 reflection coefficient for RG1020 , 50 ohm, Wr=8 mils, Lr from 1
to 15 mils.
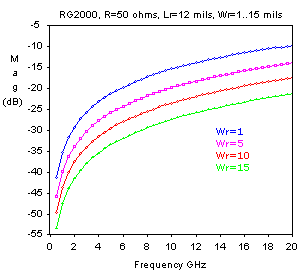
Fig.4. The S11 reflection coefficient for RG2000, 50 ohm , Lr=12 mils, Wr=1 to
15 mils.
References
1. H.Wheeler "Transmission-line Properties of a Strip on a Dielectric Sheet
on a Plane" IEEE Trans.Microwave Theory Tech. Vol.25, no.8, 1977
2. F.Schneider, W. Heinrich "Model of Thin-Film Microstripe Line for
Circuit Design" IEEE Trans.Microwave Theory Tech. Vol.49, no.1, 2001
3. A. Djordjevic, T. Sakar "Closed-Form Formulas for Frequency-Dependent
Resistance and Inductance per Unit Length of Microstrip and Strip Transmission
Line" IEEE Trans.Microwave Theory Tech. Vol.42, no.2, 1994
4. E. Hammerstad, O. Jensen "Accurate Model for Microstripe
Computer-aided Design" IEEE MTT-S Int.Microwave Symp.Dig. 1980, pp.407-409
5. W. Heinrich "Full-wave Analysis of Conductor Losses on MMIC
Transmission Lines" IEEE Trans.Microwave Theory Tech. Vol.38, no.10, 1990
|